Before we get onto the next technique, I have an apology to make. I set you on an impossible task at the end of the last post, suggesting that there might be a hidden single in 2,7 which of course was complete nonsense! Oh well….my long road to being an “expert” sudoku solver just got a bit longer.
Ignoring me and looking at the grid closer would have shown you several real hidden singles. There was a 2 in 4,2 and another in 7,7 and just spotting these two will have opened up many more. After that, the puzzle can be completed just using naked and hidden singles.
The next technique for the sudoku solver again relies on some of the material we have already covered. I have chosen to use the name block and line interactions for this one but you can find many other references to “candidate lines” and other forms of “forcing” if you look around.
If I try to analyze my own style of solving sudoku, this plays a big role in my early steps for clearing the board and I feel it works well if you are trying to get away without using pencilmarks at all when you start. I’m not a purist by any means, but if confronted by a sudoku in a newspaper, I am not tempted to mark out all the candidates straight away. So, let’s start with a new grid.
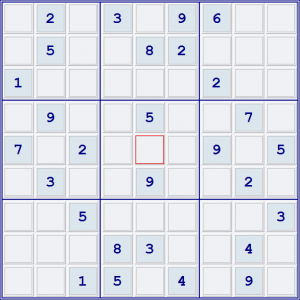
The main concept here is to try to envisage all the lines of influence made by individual numbers. Let’s look at an example to see how this works.
In the grid cells 3,1 and 9,3 there is already a given of 1. These two cells spread there one-ness (if you will) up and down the grid – and of course across and in their boxes too but these are less important for this example. As you can see, this influence affects a lot of the cells in box 4 (middle left). In fact, it only leaves one cell, right in the middle, where the 1 can now go.

Now keep looking at the same box. This time, check out the 5’s both in the rows and columns which can influence that box. Looking across, the 5’s “cancel out” both of the top two lines of our box. Similarly the 5’s above and below make it impossible for a 5 to go in the two right hand columns of our box. That just leaves the bottom left corner – cell 6,1 for the 5 to fill.
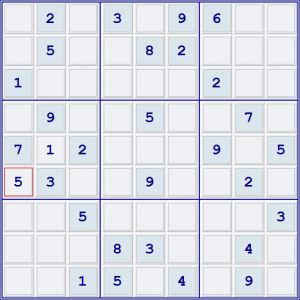
Now of course, the first step in this process is usually to try to find the number with the biggest presence on the grid already….that way there are more chances for intersections but as usual, work your way through the numbers and then go round again.
